Shooting long range at an angle is not what you think.
I just watched a video on a long range shooting page on Facebook. The guy doing the video is some kind of former Marine super sniper. Big surprise, right? Everybody who has served in the military in the past decade claims to be a sniper or a SEAL. Who the hell are driving the trucks, cooking the food, cleaning the latrines or repairing the vehicles? When everyone is Special Forces, a sniper, SEAL, or a black-ops ninja, who does the day to day work of the military?
Anyway, he is explaining shooting at long range from an elevated position. He lays out a very nice video, well spoken, well explained on a white board. The trouble is, he is wrong.
He talks about what he calls the “flat line distance.” This is a very common mistake with shooters and it’s been around for years. Heck I even wrote about it in one of my early books. The trouble is, it’s wrong and I am surprised to see a so- called military trained sniper promoting it.
The flat line distance is the distance a bullet travels in relation to the earth’s center. So when shooting from an elevated position you would draw an imaginary line from the shooter down to the same level as the target. Then another straight line to the target. This last line is the flat line distance, or the distance to the target level with the earth. The idea is that you must figure the bullet’s drop over that distance rather than the true distance the bullet will travel to the target. That’s said to be the bullet drop you will have for the much longer shot from the high angle. The idea is that you figure out how far the bullet travels relative to the core of the earth and the pull of gravity. So even if the bullet is traveling a longer distance through the air, it is affected by gravity only by the distance it is covering relative to the earth.
The science is all wrong, but the amazing thing is it all works out pretty well in terms of hitting the target, so this myth has been perpetuated for years by lots of shooters and, as I said, even by me. That’s because I researched the issue when I wrote that book almost thirty years ago and everything I found at the time, including information from a very well respected data book, said that’s how it works. Except when you think about it a little, it can’t work that way.
The theory is the bullet is traveling the flat line distance in relation to the force of gravity, so that’s our actual drop. But, gravity doesn’t work that way. Gravity is constant and it has a rate of acceleration of 32 feet per second-per second. (No, that’s not redundant as one idiot editor said after he changed it in my copy some years ago.)
That is, of course, in a vacuum. There are other forces at work, such as air friction, but they are insignificant for what we are discussing here and we can ignore them for now.
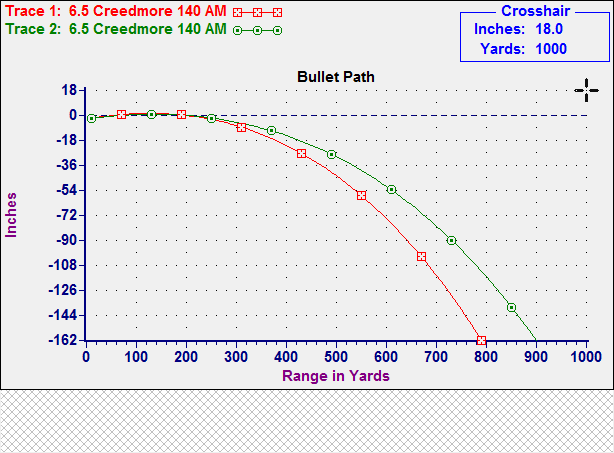
As soon as a bullet leaves the support of the rifle’s barrel it begins dropping to the earth at a constantly increasing rate of speed. The longer the bullet is in flight, the faster is it dropping. So, the key factor influencing how far the bullet actually drops is time of flight, which is when the bullet is in the air and gravity is influencing its position. That is fixed, it is physics and it does not change. The time of flight to a target is determined by the distance to the target, the velocity of the bullet and the drag factors that are slowing the velocity of the bullet. If you shoot 500 yards over flat ground it takes the same amount of time for the bullet to reach the target as it takes for a 500 yard shot from an angle. Well, almost. When shooting uphill the bullet is fighting gravity a bit more, so there is slightly more bullet drop due to a small increase in drag deceleration of the bullet, and when shooting downhill the opposite happens. Also, in both cases, if the bullet is traveling through great changes in elevation the density of the air will change and that has an effect on the friction drag on the bullet. For rifle bullets, all of these factors are small and for the point of this discussion can be ignored. For example with a 6.5 Creedmoor 140-grain bullet with a MV of 2,700 ft/s and a B.C. of .585 the difference in time of flight for 500 yards between level and a 45 degree angle is .00094 seconds. So with any given distance to the target the bullet will have (almost) the same time in flight. That means the bullet will have the same amount of drop over a given distance.

What changes are the angles and how the bullet drop relates to the line of sight. Remember line of sight is straight. If the shot is level, the bullet drops at a 90 degree angle relative to the line of sight. More or less. As explained later, the bore will not be parallel to the line of sight. But, again, for simplicity, we will call it 90 degrees. If the shot is at a steep angle, the bullet is dropping at a much different angle relative to the line of sight. Think of it this way. Stretch a string perfectly level. Now hang another string with a weight from it. The angle will be 90 degrees. Now tilt the first string to a steep angle and the distance and angle between the string and the weight will change.
The bullet is dropping at a known rate of acceleration the entire time it is in flight. If the line of sight and the bore of the rifle were parallel, the bullet would never cross the line of sight. So the bore is angled slightly muzzle up relative to the line of sight. The bullet starts below the line of sight, crosses it at a relatively short distance, then it is above the line of sight until it reaches the pre-determined zero range where it crosses the line of sight again. Beyond that distance, the bullet is always below the line of sight.
When we sight in our rifles it is almost always on a flat range where we are shooting parallel to the earth. So we have adjusted the arc of the bullet to coincide with our line of sight at the chosen zero distance. When we elevate the angle of the shot that all changes. The bullet is still dropping the same distance over a given time of flight, but the angle of how that relates to our line of sight has changed.

Same load, same distance, same zero. The green line is from a 45 degree angle while the red is shooting level.
It’s all trigonometry and confusing as hell, but it’s real. It has nothing to do with the “flat line distance,” or the “distance the bullet travels relative to the center of the earth.”
The laws of gravity are fixed, the bullet is still dropping to the earth at a rate of acceleration of 32 feet per second-per second no matter what angle you are shooting from. What has changed is the angle or the line of sight relative to the bullet’s path. This makes the shot impact higher than expected, both when shooting up and when shooting down.
The key to hitting the target is found in doing the math, using the proper cosine to figure the hold or dial up, or let one of the ballistic programs or apps handle it. What you don’t need to worry about is the “flat line distance” to the target.